[ Room 309 ]

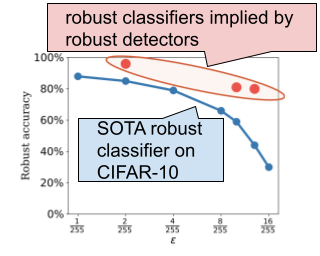
Two central problems in Stochastic Optimization are Min-Sum Set Cover and Pandora’s Box. In Pandora’s Box, we are presented with n boxes, each containing an unknown value and the goal is to open the boxes in some order to minimize the sum of the search cost and the smallest value found. Given a distribution of value vectors, we are asked to identify a near-optimal search order. Min-Sum Set Cover corresponds to the case where values are either 0 or infinity.In this work, we study the case where the value vectors are not drawn from a distribution but are presented to a learner in an online fashion. We present a computationally efficient algorithm that is constant-competitive against the cost of the optimal search order. We extend our results to a bandit setting where only the values of the boxes opened are revealed to the learner after every round. We also generalize our results to other commonly studied variants of Pandora’s Box and Min-Sum Set Cover that involve selecting more than a single value subject to a matroid constraint.
[ Hall G ]
[ Room 307 ]

Despite the broad use of fixed-point iterations throughout applied mathematics, the optimal convergence rate of general fixed-point problems with nonexpansive nonlinear operators has not been established. This work presents an acceleration mechanism for fixed-point iterations with nonexpansive operators, contractive operators, and nonexpansive operators satisfying a H\"older-type growth condition. We then provide matching complexity lower bounds to establish the exact optimality of the acceleration mechanisms in the nonexpansive and contractive setups. Finally, we provide experiments with CT imaging, optimal transport, and decentralized optimization to demonstrate the practical effectiveness of the acceleration mechanism.
[ Ballroom 3 & 4 ]

Bayesian neural networks (BNNs) promise improved generalization under covariate shift by providing principled probabilistic representations of epistemic uncertainty. However, weight-based BNNs often struggle with high computational complexity of large-scale architectures and datasets. Node-based BNNs have recently been introduced as scalable alternatives, which induce epistemic uncertainty by multiplying each hidden node with latent random variables, while learning a point-estimate of the weights. In this paper, we interpret these latent noise variables as implicit representations of simple and domain-agnostic data perturbations during training, producing BNNs that perform well under covariate shift due to input corruptions. We observe that the diversity of the implicit corruptions depends on the entropy of the latent variables, and propose a straightforward approach to increase the entropy of these variables during training. We evaluate the method on out-of-distribution image classification benchmarks, and show improved uncertainty estimation of node-based BNNs under covariate shift due to input perturbations. As a side effect, the method also provides robustness against noisy training labels.
[ Room 301 - 303 ]

[ Hall F ]
[ Room 327 - 329 ]
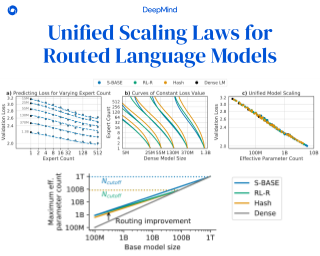
The performance of a language model has been shown to be effectively modeled as a power-law in its parameter count. Here we study the scaling behaviors of Routing Networks: architectures that conditionally use only a subset of their parameters while processing an input. For these models, parameter count and computational requirement form two independent axes along which an increase leads to better performance. In this work we derive and justify scaling laws defined on these two variables which generalize those known for standard language models and describe the performance of a wide range of routing architectures trained via three different techniques. Afterwards we provide two applications of these laws: first deriving an Effective Parameter Count along which all models scale at the same rate, and then using the scaling coefficients to give a quantitative comparison of the three routing techniques considered. Our analysis derives from an extensive evaluation of Routing Networks across five orders of magnitude of size, including models with hundreds of experts and hundreds of billions of parameters.
[ Room 310 ]
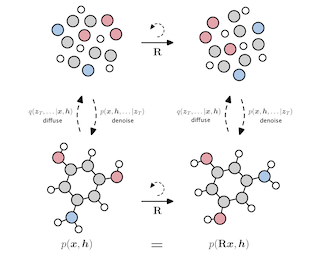
This work introduces a diffusion model for molecule generation in 3D that is equivariant to Euclidean transformations. Our E(3) Equivariant Diffusion Model (EDM) learns to denoise a diffusion process with an equivariant network that jointly operates on both continuous (atom coordinates) and categorical features (atom types). In addition, we provide a probabilistic analysis which admits likelihood computation of molecules using our model. Experimentally, the proposed method significantly outperforms previous 3D molecular generative methods regarding the quality of generated samples and the efficiency at training time.
[ Room 318 - 320 ]
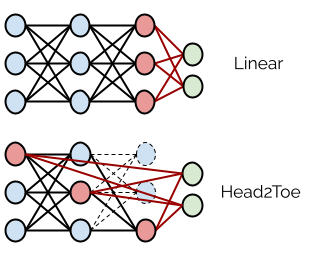
Transfer-learning methods aim to improve performance in a data-scarce target domain using a model pretrained on a data-rich source domain. A cost-efficient strategy, linear probing, involves freezing the source model and training a new classification head for the target domain. This strategy is outperformed by a more costly but state-of-the-art method -- fine-tuning all parameters of the source model to the target domain -- possibly because fine-tuning allows the model to leverage useful information from intermediate layers which is otherwise discarded by the later previously trained layers. We explore the hypothesis that these intermediate layers might be directly exploited. We propose a method, Head-to-Toe probing (Head2Toe), that selects features from all layers of the source model to train a classification head for the target-domain. In evaluations on the Visual Task Adaptation Benchmark-1k, Head2Toe matches performance obtained with fine-tuning on average while reducing training and storage cost hundred folds or more, but critically, for out-of-distribution transfer, Head2Toe outperforms fine-tuning. Code used in our experiments can be found in supplementary materials.
[ Ballroom 1 & 2 ]
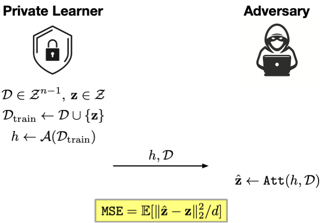
Differential privacy is widely accepted as the de facto method for preventing data leakage in ML, and conventional wisdom suggests that it offers strong protection against privacy attacks. However, existing semantic guarantees for DP focus on membership inference, which may overestimate the adversary's capabilities and is not applicable when membership status itself is non-sensitive. In this paper, we derive the first semantic guarantees for DP mechanisms against training data reconstruction attacks under a formal threat model. We show that two distinct privacy accounting methods---Renyi differential privacy and Fisher information leakage---both offer strong semantic protection against data reconstruction attacks.
[ Ballroom 3 & 4 ]
Bayesian structure learning allows one to capture uncertainty over the causal directed acyclic graph (DAG) responsible for generating given data. In this work, we present Tractable Uncertainty for STructure learning (TRUST), a framework for approximate posterior inference that relies on probabilistic circuits as a representation of our posterior belief. In contrast to sample-based posterior approximations, our representation can capture a much richer space of DAGs, while being able to tractably answer a range of useful inference queries. We empirically demonstrate how probabilistic circuits can be used to as an augmented representation for structure learning methods, leading to improvement in both the quality of inferred structures and posterior uncertainty. Experimental results also demonstrate the improved representational capacity of TRUST, outperforming competing methods on conditional query answering.
[ Hall G ]

Label smoothing (LS) is an arising learning paradigm that uses the positively weighted average of both the hard training labels and uniformly distributed soft labels. It was shown that LS serves as a regularizer for training data with hard labels and therefore improves the generalization of the model. Later it was reported LS even helps with improving robustness when learning with noisy labels. However, we observed that the advantage of LS vanishes when we operate in a high label noise regime. Intuitively speaking, this is due to the increased entropy of P(noisy label|X) when the noise rate is high, in which case, further applying LS tends to “over-smooth” the estimated posterior. We proceeded to discover that several learning-with-noisy-labels solutions in the literature instead relate more closely to negative/not label smoothing (NLS), which acts counter to LS and defines as using a negative weight to combine the hard and soft labels! We provide understandings for the properties of LS and NLS when learning with noisy labels. Among other established properties, we theoretically show NLS is considered more beneficial when the label noise rates are high. We provide extensive experimental results on multiple benchmarks to support our findings too. Code is publicly …
[ Room 309 ]

The K-armed dueling bandit problem, where the feedback is in the form of noisy pairwise comparisons, has been widely studied. Previous works have only focused on the sequential setting where the policy adapts after every comparison. However, in many applications such as search ranking and recommendation systems, it is preferable to perform comparisons in a limited number of parallel batches. We study the batched K-armed dueling bandit problem under two standard settings: (i) existence of a Condorcet winner, and (ii) strong stochastic transitivity and stochastic triangle inequality. For both settings, we obtain algorithms with a smooth trade-off between the number of batches and regret. Our regret bounds match the best known sequential regret bounds (up to poly-logarithmic factors), using only a logarithmic number of batches. We complement our regret analysis with a nearly-matching lower bound. Finally, we also validate our theoretical results via experiments on synthetic and real data.
[ Room 307 ]

[ Room 309 ]
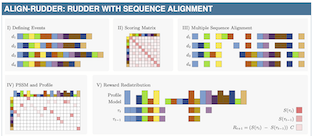
Reinforcement learning algorithms require many samples when solving complex hierarchical tasks with sparse and delayed rewards. For such complex tasks, the recently proposed RUDDER uses reward redistribution to leverage steps in the Q-function that are associated with accomplishing sub-tasks. However, often only few episodes with high rewards are available as demonstrations since current exploration strategies cannot discover them in reasonable time. In this work, we introduce Align-RUDDER, which utilizes a profile model for reward redistribution that is obtained from multiple sequence alignment of demonstrations. Consequently, Align-RUDDER employs reward redistribution effectively and, thereby, drastically improves learning on few demonstrations. Align-RUDDER outperforms competitors on complex artificial tasks with delayed rewards and few demonstrations. On the Minecraft ObtainDiamond task, Align-RUDDER is able to mine a diamond, though not frequently. Code is available at github.com/ml-jku/align-rudder.
[ Room 318 - 320 ]

Model compression is an essential technique for deploying deep neural networks (DNNs) on power and memory-constrained resources. However, existing model-compression methods often rely on human expertise and focus on parameters' local importance, ignoring the rich topology information within DNNs. In this paper, we propose a novel multi-stage graph embedding technique based on graph neural networks (GNNs) to identify DNN topologies and use reinforcement learning (RL) to find a suitable compression policy. We performed resource-constrained (i.e., FLOPs) channel pruning and compared our approach with state-of-the-art model compression methods.We evaluated our method on various models from typical to mobile-friendly networks, such as ResNet family, VGG-16, MobileNet-v1/v2, and ShuffleNet. Results show that our method can achieve higher compression ratios with a minimal fine-tuning cost yet yields outstanding and competitive performance.
[ Hall G ]

[ Room 310 ]

This paper proves that robustness implies generalization via data-dependent generalization bounds. As a result, robustness and generalization are shown to be connected closely in a data-dependent manner. Our bounds improve previous bounds in two directions, to solve an open problem that has seen little development since 2010. The first is to reduce the dependence on the covering number. The second is to remove the dependence on the hypothesis space. We present several examples, including ones for lasso and deep learning, in which our bounds are provably preferable. The experiments on real-world data and theoretical models demonstrate near-exponential improvements in various situations. To achieve these improvements, we do not require additional assumptions on the unknown distribution; instead, we only incorporate an observable and computable property of the training samples. A key technical innovation is an improved concentration bound for multinomial random variables that is of independent interest beyond robustness and generalization.
[ Ballroom 1 & 2 ]
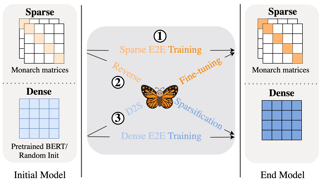
Large neural networks excel in many domains, but they are expensive to train and fine-tune. A popular approach to reduce their compute or memory requirements is to replace dense weight matrices with structured ones (e.g., sparse, low-rank, Fourier transform). These methods have not seen widespread adoption (1) in end-to-end training due to unfavorable efficiency--quality tradeoffs, and (2) in dense-to-sparse fine-tuning due to lack of tractable algorithms to approximate a given dense weight matrix. To address these issues, we propose a class of matrices (Monarch) that is hardware-efficient (they are parameterized as products of two block-diagonal matrices for better hardware utilization) and expressive (they can represent many commonly used transforms). Surprisingly, the problem of approximating a dense weight matrix with a Monarch matrix, though nonconvex, has an analytical optimal solution. These properties of Monarch matrices unlock new ways to train and fine-tune sparse and dense models. We empirically validate that Monarch can achieve favorable accuracy-efficiency tradeoffs in several end-to-end sparse training applications: speeding up ViT and GPT-2 training on ImageNet classification and Wikitext-103 language modeling by 2x with comparable model quality, and reducing the error on PDE solving and MRI reconstruction tasks by 40%. In sparse-to-dense training, with a simple technique …
[ Room 301 - 303 ]

[ Hall F ]
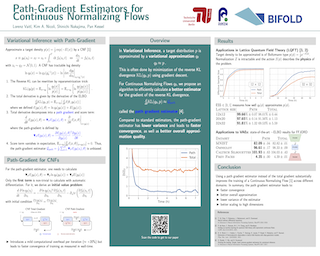
Recent work has established a path-gradient estimator for simple variational Gaussian distributions and has argued that the path-gradient is particularly beneficial in the regime in which the variational distribution approaches the exact target distribution. In many applications, this regime can however not be reached by a simple Gaussian variational distribution. In this work, we overcome this crucial limitation by proposing a path-gradient estimator for the considerably more expressive variational family of continuous normalizing flows. We outline an efficient algorithm to calculate this estimator and establish its superior performance empirically.
[ Room 307 ]
Gaussian process hyperparameter optimization requires linear solves with, and log-determinants of, large kernel matrices. Iterative numerical techniques are becoming popular to scale to larger datasets, relying on the conjugate gradient method (CG) for the linear solves and stochastic trace estimation for the log-determinant. This work introduces new algorithmic and theoretical insights for preconditioning these computations. While preconditioning is well understood in the context of CG, we demonstrate that it can also accelerate convergence and reduce variance of the estimates for the log-determinant and its derivative. We prove general probabilistic error bounds for the preconditioned computation of the log-determinant, log-marginal likelihood and its derivatives. Additionally, we derive specific rates for a range of kernel-preconditioner combinations, showing that up to exponential convergence can be achieved. Our theoretical results enable provably efficient optimization of kernel hyperparameters, which we validate empirically on large-scale benchmark problems. There our approach accelerates training by up to an order of magnitude.
[ Room 327 - 329 ]

While the general idea of self-supervised learning is identical across modalities, the actual algorithms and objectives differ widely because they were developed with a single modality in mind. To get us closer to general self-supervised learning, we present data2vec, a framework that uses the same learning method for either speech, NLP or computer vision. The core idea is to predict latent representations of the full input data based on a masked view of the input in a self-distillation setup using a standard Transformer architecture. Instead of predicting modality-specific targets such as words, visual tokens or units of human speech which are local in nature, data2vec predicts contextualized latent representations that contain information from the entire input. Experiments on the major benchmarks of speech recognition, image classification, and natural language understanding demonstrate a new state of the art or competitive performance to predominant approaches.
[ Ballroom 3 & 4 ]
Characteristic functions (from cooperative game theory) are able to evaluate partial inputs and form the basis for attribution methods like Shapley values. These attribution methods allow us to measure how important each input component is for the function output---one of the goals of explainable AI (XAI).Given a standard classifier function, it is unclear how partial input should be realised.Instead, most XAI-methods for black-box classifiers like neural networks consider counterfactual inputs that generally lie off-manifold, which makes them hard to evaluate and easy to manipulate.We propose a setup to directly train characteristic functions in the form of neural networks to play simple two-player games. We apply this to the game of Connect Four by randomly hiding colour information from our agents during training. This has three advantages for comparing XAI-methods: It alleviates the ambiguity about how to realise partial input, makes off-manifold evaluation unnecessary and allows us to compare the methods by letting them play against each other.
[ Room 318 - 320 ]

[ Hall G ]
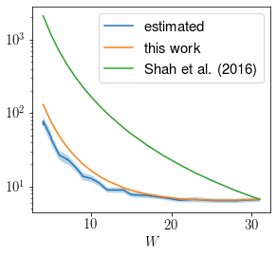
[ Room 310 ]
In this paper, we propose a natural notion of individual preference (IP) stability for clustering, which asks that every data point, on average, is closer to the points in its own cluster than to the points in any other cluster. Our notion can be motivated from several perspectives, including game theory and algorithmic fairness. We study several questions related to our proposed notion. We first show that deciding whether a given data set allows for an IP-stable clustering in general is NP-hard. As a result, we explore the design of efficient algorithms for finding IP-stable clusterings in some restricted metric spaces. We present a polytime algorithm to find a clustering satisfying exact IP-stability on the real line, and an efficient algorithm to find an IP-stable 2-clustering for a tree metric. We also consider relaxing the stability constraint, i.e., every data point should not be too far from its own cluster compared to any other cluster. For this case, we provide polytime algorithms with different guarantees. We evaluate some of our algorithms and several standard clustering approaches on real data sets.
[ Room 309 ]
Offline RL algorithms must account for the fact that the dataset they are provided may leave many facets of the environment unknown. The most common way to approach this challenge is to employ pessimistic or conservative methods, which avoid behaviors that are too dissimilar from those in the training dataset. However, relying exclusively on conservatism has drawbacks: performance is sensitive to the exact degree of conservatism, and conservative objectives can recover highly suboptimal policies. In this work, we propose that offline RL methods should instead be adaptive in the presence of uncertainty. We show that acting optimally in offline RL in a Bayesian sense involves solving an implicit POMDP. As a result, optimal policies for offline RL must be adaptive, depending not just on the current state but rather all the transitions seen so far during evaluation. We present a model-free algorithm for approximating this optimal adaptive policy, and demonstrate the efficacy of learning such adaptive policies in offline RL benchmarks.
[ Room 307 ]
[ Hall F ]

Out-of-distribution (OOD) detection is indispensable for machine learning models deployed in the open world. Recently, the use of an auxiliary outlier dataset during training (also known as outlier exposure) has shown promising performance. As the sample space for potential OOD data can be prohibitively large, sampling informative outliers is essential. In this work, we propose a novel posterior sampling based outlier mining framework, POEM, which facilitates efficient use of outlier data and promotes learning a compact decision boundary between ID and OOD data for improved detection. We show that POEM establishes state-of-the-art performance on common benchmarks. Compared to the current best method that uses a greedy sampling strategy, POEM improves the relative performance by 42.0% and 24.2% (FPR95) on CIFAR-10 and CIFAR-100, respectively. We further provide theoretical insights on the effectiveness of POEM for OOD detection.
[ Room 310 ]
[ Hall G ]
[ Room 301 - 303 ]

Learning meaningful representations of data that can address challenges such as batch effect correction and counterfactual inference is a central problem in many domains including computational biology. Adopting a Conditional VAE framework, we show that marginal independence between the representation and a condition variable plays a key role in both of these challenges. We propose the Contrastive Mixture of Posteriors (CoMP) method that uses a novel misalignment penalty defined in terms of mixtures of the variational posteriors to enforce this independence in latent space. We show that CoMP has attractive theoretical properties compared to previous approaches, and we prove counterfactual identifiability of CoMP under additional assumptions. We demonstrate state-of-the-art performance on a set of challenging tasks including aligning human tumour samples with cancer cell-lines, predicting transcriptome-level perturbation responses, and batch correction on single-cell RNA sequencing data. We also find parallels to fair representation learning and demonstrate that CoMP is competitive on a common task in the field.
[ Room 327 - 329 ]

Neural Ordinary Differential Equations model dynamical systems with ODEs learned by neural networks.However, ODEs are fundamentally inadequate to model systems with long-range dependencies or discontinuities, which are common in engineering and biological systems. Broader classes of differential equations (DE) have been proposed as remedies, including delay differential equations and integro-differential equations.Furthermore, Neural ODE suffers from numerical instability when modelling stiff ODEs and ODEs with piecewise forcing functions.In this work, we propose Neural Laplace, a unifying framework for learning diverse classes of DEs including all the aforementioned ones.Instead of modelling the dynamics in the time domain, we model it in the Laplace domain, where the history-dependencies and discontinuities in time can be represented as summations of complex exponentials. To make learning more efficient, we use the geometrical stereographic map of a Riemann sphere to induce more smoothness in the Laplace domain.In the experiments, Neural Laplace shows superior performance in modelling and extrapolating the trajectories of diverse classes of DEs, including the ones with complex history dependency and abrupt changes.
[ Room 318 - 320 ]

[ Room 309 ]
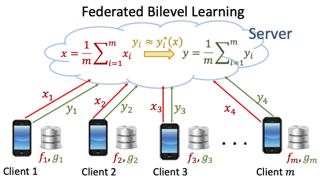
Standard federated optimization methods successfully apply to stochastic problems with single-level structure. However, many contemporary ML problems - including adversarial robustness, hyperparameter tuning, actor-critic - fall undernested bilevel programming that subsumes minimax and compositional optimization. In this work, we propose FedNest: A federated alternating stochastic gradient method to address general nested problems. We establish provable convergence rates for FedNest in the presence of heterogeneous data and introduce variations for bilevel, minimax, and compositional optimization. FedNest introduces multiple innovations including federated hypergradient computation and variance reduction to address inner-level heterogeneity. We complement our theory with experiments on hyperparameter & hyper-representation learning and minimax optimization that demonstrate the benefits of our method in practice.
[ Ballroom 3 & 4 ]

Pearl’s do calculus is a complete axiomatic approach to learn the identifiable causal effects from observational data. When such an effect is not identifiable, it is necessary to perform a collection of often costly interventions in the system to learn the causal effect. In this work, we consider the problem of designing the collection of interventions with the minimum cost to identify the desired effect. First, we prove that this prob-em is NP-complete, and subsequently propose an algorithm that can either find the optimal solution or a logarithmic-factor approximation of it. This is done by establishing a connection between our problem and the minimum hitting set problem. Additionally, we propose several polynomial time heuristic algorithms to tackle the computational complexity of the problem. Although these algorithms could potentially stumble on sub-optimal solutions, our simulations show that they achieve small regrets on random graphs.
[ Ballroom 1 & 2 ]
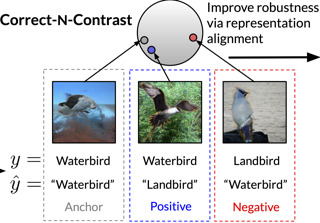
Spurious correlations pose a major challenge for robust machine learning. Models trained with empirical risk minimization (ERM) may learn to rely on correlations between class labels and spurious attributes, leading to poor performance on data groups without these correlations. This is challenging to address when the spurious attribute labels are unavailable. To improve worst-group performance on spuriously correlated data without training attribute labels, we propose Correct-N-Contrast (CNC), a contrastive approach to directly learn representations robust to spurious correlations. As ERM models can be good spurious attribute predictors, CNC works by (1) using a trained ERM model’s outputs to identify samples with the same class but dissimilar spurious features, and (2) training a robust model with contrastive learning to learn similar representations for these samples. To support CNC, we introduce new connections between worst-group error and a representation alignment loss that CNC aims to minimize. We empirically observe that worst-group error closely tracks with alignment loss, and prove that the alignment loss over a class helps upper-bound the class's worst-group vs. average error gap. On popular benchmarks, CNC reduces alignment loss drastically, and achieves state-of-the-art worst-group accuracy by 3.6% average absolute lift. CNC is also competitive with oracle methods that require …
[ Hall F ]

As real-world images come in varying sizes, the machine learning model is part of a larger system that includes an upstream image scaling algorithm. In this paper, we investigate the interplay between vulnerabilities of the image scaling procedure and machine learning models in the decision-based black-box setting. We propose a novel sampling strategy to make a black-box attack exploit vulnerabilities in scaling algorithms, scaling defenses, and the final machine learning model in an end-to-end manner. Based on this scaling-aware attack, we reveal that most existing scaling defenses are ineffective under threat from downstream models. Moreover, we empirically observe that standard black-box attacks can significantly improve their performance by exploiting the vulnerable scaling procedure. We further demonstrate this problem on a commercial Image Analysis API with decision-based black-box attacks.
[ Room 310 ]

Designing efficient general-purpose contextual bandit algorithms that work with large---or even infinite---action spaces would facilitate application to important scenarios such as information retrieval, recommendation systems, and continuous control. While obtaining standard regret guarantees can be hopeless, alternative regret notions have been proposed to tackle the large action setting. We propose a smooth regret notion for contextual bandits, which dominates previously proposed alternatives. We design a statistically and computationally efficient algorithm---for the proposed smooth regret---that works with general function approximation under standard supervised oracles. We also present an adaptive algorithm that automatically adapts to any smoothness level. Our algorithms can be used to recover the previous minimax/Pareto optimal guarantees under the standard regret, e.g., in bandit problems with multiple best arms and Lipschitz/H{\"o}lder bandits. We conduct large-scale empirical evaluations demonstrating the efficacy of our proposed algorithms.
[ Room 307 ]

A challenging problem in probabilistic programming is to develop inference algorithms that work for arbitrary programs in a universal probabilistic programming language (PPL). We present the nonparametric involutive Markov chain Monte Carlo (NP-iMCMC) algorithm as a method for constructing MCMC inference algorithms for nonparametric models expressible in universal PPLs. Building on the unifying involutive MCMC framework, and by providing a general procedure for driving state movement between dimensions, we show that NP-iMCMC can generalise numerous existing iMCMC algorithms to work on nonparametric models. We prove the correctness of the NP-iMCMC sampler. Our empirical study shows that the existing strengths of several iMCMC algorithms carry over to their nonparametric extensions. Applying our method to the recently proposed Nonparametric HMC, an instance of (Multiple Step) NP-iMCMC, we have constructed several nonparametric extensions (all of which new) that exhibit significant performance improvements.
[ Hall G ]

We revisit the classical problem of deriving convergence rates for the maximum likelihood estimator (MLE) in finite mixture models. The Wasserstein distance has become a standard loss function for the analysis of parameter estimation in these models, due in part to its ability to circumvent label switching and to accurately characterize the behaviour of fitted mixture components with vanishing weights. However, the Wasserstein distance is only able to capture the worst-case convergence rate among the remaining fitted mixture components. We demonstrate that when the log-likelihood function is penalized to discourage vanishing mixing weights, stronger loss functions can be derived to resolve this shortcoming of the Wasserstein distance. These new loss functions accurately capture the heterogeneity in convergence rates of fitted mixture components, and we use them to sharpen existing pointwise and uniform convergence rates in various classes of mixture models. In particular, these results imply that a subset of the components of the penalized MLE typically converge significantly faster than could have been anticipated from past work. We further show that some of these conclusions extend to the traditional MLE. Our theoretical findings are supported by a simulation study to illustrate these improved convergence rates.
[ Room 327 - 329 ]
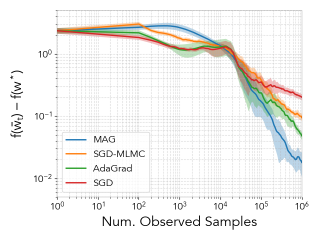
We consider stochastic optimization problems where data is drawn from a Markov chain. Existing methods for this setting crucially rely on knowing the mixing time of the chain, which in real-world applications is usually unknown. We propose the first optimization method that does not require the knowledge of the mixing time, yet obtains the optimal asymptotic convergence rate when applied to convex problems. We further show that our approach can be extended to: (i) finding stationary points in non-convex optimization with Markovian data, and (ii) obtaining better dependence on the mixing time in temporal difference (TD) learning; in both cases, our method is completely oblivious to the mixing time. Our method relies on a novel combination of multi-level Monte Carlo (MLMC) gradient estimation together with an adaptive learning method.
[ Room 310 ]
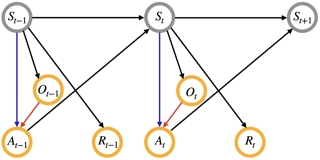
We consider off-policy evaluation (OPE) in Partially Observable Markov Decision Processes (POMDPs), where the evaluation policy depends only on observable variables and the behavior policy depends on unobservable latent variables. Existing works either assume no unmeasured confounders, or focus on settings where both the observation and the state spaces are tabular. In this work, we first propose novel identification methods for OPE in POMDPs with latent confounders, by introducing bridge functions that link the target policy's value and the observed data distribution. We next propose minimax estimation methods for learning these bridge functions, and construct three estimators based on these estimated bridge functions, corresponding to a value function-based estimator, a marginalized importance sampling estimator, and a doubly-robust estimator. Our proposal permits general function approximation and is thus applicable to settings with continuous or large observation/state spaces. The nonasymptotic and asymptotic properties of the proposed estimators are investigated in detail. A Python implementation of our proposal is available at https://github.com/jiaweihhuang/ Confounded-POMDP-Exp.
[ Room 301 - 303 ]

While Bayesian neural networks (BNNs) provide a sound and principled alternative to standard neural networks, an artificial sharpening of the posterior usually needs to be applied to reach comparable performance. This is in stark contrast to theory, dictating that given an adequate prior and a well-specified model, the untempered Bayesian posterior should achieve optimal performance. Despite the community's extensive efforts, the observed gains in performance still remain disputed with several plausible causes pointing at its origin. While data augmentation has been empirically recognized as one of the main drivers of this effect, a theoretical account of its role, on the other hand, is largely missing. In this work we identify two interlaced factors concurrently influencing the strength of the cold posterior effect, namely the correlated nature of augmentations and the degree of invariance of the employed model to such transformations. By theoretically analyzing simplified settings, we prove that tempering implicitly reduces the misspecification arising from modeling augmentations as i.i.d. data. The temperature mimics the role of the effective sample size, reflecting the gain in information provided by the augmentations. We corroborate our theoretical findings with extensive empirical evaluations, scaling to realistic BNNs. By relying on the framework of group convolutions, …
[ Hall F ]
We study covariate shift in the context of nonparametric regression. We introduce a new measure of distribution mismatch between the source and target distributions using the integrated ratio of probabilities of balls at a given radius. We use the scaling of this measure with respect to the radius to characterize the minimax rate of estimation over a family of Hölder continuous functions under covariate shift. In comparison to the recently proposed notion of transfer exponent, this measure leads to a sharper rate of convergence and is more fine-grained. We accompany our theory with concrete instances of covariate shift that illustrate this sharp difference.
[ Room 318 - 320 ]
[ Room 307 ]
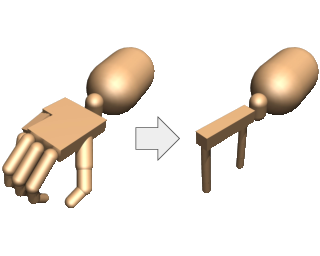
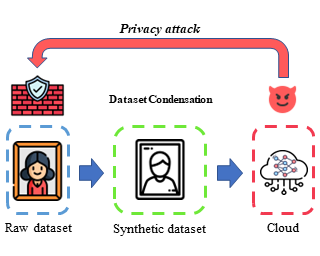
A popular paradigm in robotic learning is to train a policy from scratch for every new robot. This is not only inefficient but also often impractical for complex robots. In this work, we consider the problem of transferring a policy across two different robots with significantly different parameters such as kinematics and morphology. Existing approaches that train a new policy by matching the action or state transition distribution, including imitation learning methods, fail due to optimal action and/or state distribution being mismatched in different robots. In this paper, we propose a novel method named REvolveR of using continuous evolutionary models for robotic policy transfer implemented in a physics simulator. We interpolate between the source robot and the target robot by finding a continuous evolutionary change of robot parameters. An expert policy on the source robot is transferred through training on a sequence of intermediate robots that gradually evolve into the target robot. Experiments on a physics simulator show that the proposed continuous evolutionary model can effectively transfer the policy across robots and achieve superior sample efficiency on new robots. The proposed method is especially advantageous in sparse reward settings where exploration can be significantly reduced.
[ Ballroom 3 & 4 ]
[ Room 309 ]
[ Hall G ]
We consider the problem of predicting a protein sequence from its backbone atom coordinates. Machine learning approaches to this problem to date have been limited by the number of available experimentally determined protein structures. We augment training data by nearly three orders of magnitude by predicting structures for 12M protein sequences using AlphaFold2. Trained with this additional data, a sequence-to-sequence transformer with invariant geometric input processing layers achieves 51% native sequence recovery on structurally held-out backbones with 72% recovery for buried residues, an overall improvement of almost 10 percentage points over existing methods. The model generalizes to a variety of more complex tasks including design of protein complexes, partially masked structures, binding interfaces, and multiple states.
[ Ballroom 1 & 2 ]
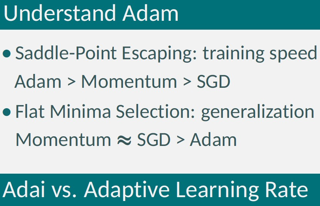
Adaptive Moment Estimation (Adam), which combines Adaptive Learning Rate and Momentum, would be the most popular stochastic optimizer for accelerating the training of deep neural networks. However, it is empirically known that Adam often generalizes worse than Stochastic Gradient Descent (SGD). The purpose of this paper is to unveil the mystery of this behavior in the diffusion theoretical framework. Specifically, we disentangle the effects of Adaptive Learning Rate and Momentum of the Adam dynamics on saddle-point escaping and flat minima selection. We prove that Adaptive Learning Rate can escape saddle points efficiently, but cannot select flat minima as SGD does. In contrast, Momentum provides a drift effect to help the training process pass through saddle points, and almost does not affect flat minima selection. This partly explains why SGD (with Momentum) generalizes better, while Adam generalizes worse but converges faster. Furthermore, motivated by the analysis, we design a novel adaptive optimization framework named Adaptive Inertia, which uses parameter-wise adaptive inertia to accelerate the training and provably favors flat minima as well as SGD. Our extensive experiments demonstrate that the proposed adaptive inertia method can generalize significantly better than SGD and conventional adaptive gradient methods.
[ Room 310 ]
[ Room 327 - 329 ]

[ Room 301 - 303 ]
Bayesian additive regression trees (BART; Chipman et al., 2010) have gained great popularity as a flexible nonparametric function estimation and modeling tool. Nearly all existing BART models rely on decision tree weak learners with axis-parallel univariate split rules to partition the Euclidean feature space into rectangular regions. In practice, however, many regression problems involve features with multivariate structures (e.g., spatial locations) possibly lying in a manifold, where rectangular partitions may fail to respect irregular intrinsic geometry and boundary constraints of the structured feature space. In this paper, we develop a new class of Bayesian additive multivariate decision tree models that combine univariate split rules for handling possibly high dimensional features without known multivariate structures and novel multivariate split rules for features with multivariate structures in each weak learner. The proposed multivariate split rules are built upon stochastic predictive spanning tree bipartition models on reference knots, which are capable of achieving highly flexible nonlinear decision boundaries on manifold feature spaces while enabling efficient dimension reduction computations. We demonstrate the superior performance of the proposed method using simulation data and a Sacramento housing price data set.
[ Room 309 ]

[ Room 301 - 303 ]
Tensor decomposition is a dominant framework for multiway data analysis and prediction. Although practical data often contains timestamps for the observed entries, existing tensor decomposition approaches overlook or under-use this valuable time information. They either drop the timestamps or bin them into crude steps and hence ignore the temporal dynamics within each step or use simple parametric time coefficients. To overcome these limitations, we propose Bayesian Continuous-Time Tucker Decomposition. We model the tensor-core of the classical Tucker decomposition as a time-varying function, and place a Gaussian process prior to flexibly estimate all kinds of temporal dynamics. In this way, our model maintains the interpretability while is flexible enough to capture various complex temporal relationships between the tensor nodes. For efficient and high-quality posterior inference, we use the stochastic differential equation (SDE) representation of temporal GPs to build an equivalent state-space prior, which avoids huge kernel matrix computation and sparse/low-rank approximations. We then use Kalman filtering, RTS smoothing, and conditional moment matching to develop a scalable message passing inference algorithm. We show the advantage of our method in simulation and several real-world applications.
[ Room 327 - 329 ]
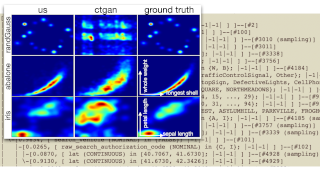
[ Room 307 ]
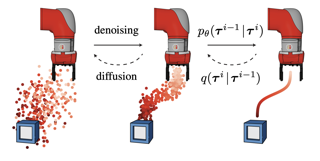
Model-based reinforcement learning methods often use learning only for the purpose of recovering an approximate dynamics model, offloading the rest of the decision-making work to classical trajectory optimizers.While conceptually simple, this combination has a number of empirical shortcomings, suggesting that learned models may not be well-suited to standard trajectory optimization.In this paper, we consider what it would look like to fold as much of the trajectory optimization pipeline as possible into the modeling problem, such that sampling from the model and planning with it become nearly identical.The core of our technical approach lies in a diffusion probabilistic model that plans by iteratively denoising trajectories.We show how classifier-guided sampling and image inpainting can be reinterpreted as coherent planning strategies, explore the unusual and useful properties of diffusion-based planning methods, and demonstrate the effectiveness of our framework in control settings that emphasize long-horizon decision-making and test-time flexibility.
[ Hall G ]

[ Hall F ]
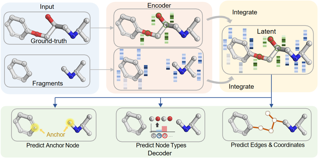
Deep learning has achieved tremendous success in designing novel chemical compounds with desirable pharmaceutical properties. In this work, we focus on a new type of drug design problem --- generating a small ``linker'' to physically attach two independent molecules with their distinct functions. The main computational challenges include: 1) the generation of linkers is conditional on the two given molecules, in contrast to generating complete molecules from scratch in previous works; 2) linkers heavily depend on the anchor atoms of the two molecules to be connected, which are not known beforehand; 3) 3D structures and orientations of the molecules need to be considered to avoid atom clashes, for which equivariance to E(3) group are necessary. To address these problems, we propose a conditional generative model, named 3DLinker, which is able to predict anchor atoms and jointly generate linker graphs and their 3D structures based on an E(3) equivariant graph variational autoencoder. So far as we know, no previous models could achieve this task. We compare our model with multiple conditional generative models modified from other molecular design tasks and find that our model has a significantly higher rate in recovering molecular graphs, and more importantly, accurately predicting the 3D coordinates …
[ Room 318 - 320 ]
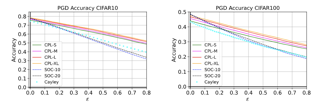
[ Room 310 ]
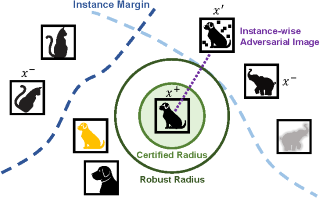
Contrastive adversarial training has successfully improved the robustness of contrastive learning (CL). However, the robustness metric used in these methods is linked to attack algorithms, image labels and downstream tasks, all of which may affect the consistency and reliability of robustness metric for CL. To address these problems, this paper proposes a novel Robustness Verification framework for Contrastive Learning (RVCL). Furthermore, we use extreme value theory to reveal the relationship between the robust radius of the CL encoder and that of the supervised downstream task. Extensive experimental results on various benchmark models and datasets verify our theoretical findings, and further demonstrate that our proposed RVCL is able to evaluate the robustness of both models and images. Our code is available at https://github.com/wzekai99/RVCL.
[ Ballroom 1 & 2 ]
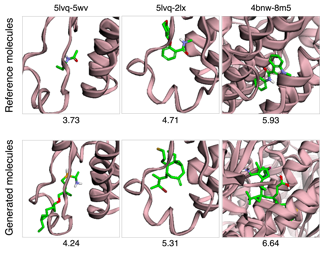
A fundamental problem in drug discovery is to design molecules that bind to specific proteins. To tackle this problem using machine learning methods, here we propose a novel and effective framework, known as GraphBP, to generate 3D molecules that bind to given proteins by placing atoms of specific types and locations to the given binding site one by one. In particular, at each step, we first employ a 3D graph neural network to obtain geometry-aware and chemically informative representations from the intermediate contextual information. Such context includes the given binding site and atoms placed in the previous steps. Second, to preserve the desirable equivariance property, we select a local reference atom according to the designed auxiliary classifiers and then construct a local spherical coordinate system. Finally, to place a new atom, we generate its atom type and relative location w.r.t. the constructed local coordinate system via a flow model. We also consider generating the variables of interest sequentially to capture the underlying dependencies among them. Experiments demonstrate that our GraphBP is effective to generate 3D molecules with binding ability to target protein binding sites. Our implementation is available at https://github.com/divelab/GraphBP.
[ Ballroom 3 & 4 ]

The fast spreading adoption of machine learning (ML) by companies across industries poses significant regulatory challenges. One such challenge is scalability: how can regulatory bodies efficiently \emph{audit} these ML models, ensuring that they are fair? In this paper, we initiate the study of query-based auditing algorithms that can estimate the demographic parity of ML models in a query-efficient manner. We propose an optimal deterministic algorithm, as well as a practical randomized, oracle-efficient algorithm with comparable guarantees. Furthermore, we make inroads into understanding the optimal query complexity of randomized active fairness estimation algorithms. Our first exploration of active fairness estimation aims to put AI governance on firmer theoretical foundations.
[ Room 309 ]

[ Room 327 - 329 ]
We investigate approximation guarantees provided by logistic regression for the fundamental problem of agnostic learning of homogeneous halfspaces. Previously, for a certain broad class of “well-behaved” distributions on the examples, Diakonikolas et al. (2020) proved an tilde{Omega}(OPT) lower bound, while Frei et al. (2021) proved an tilde{O}(sqrt{OPT}) upper bound, where OPT denotes the best zero-one/misclassification risk of a homogeneous halfspace. In this paper, we close this gap by constructing a well-behaved distribution such that the global minimizer of the logistic risk over this distribution only achieves Omega(sqrt{OPT}) misclassification risk, matching the upper bound in (Frei et al., 2021). On the other hand, we also show that if we impose a radial-Lipschitzness condition in addition to well-behaved-ness on the distribution, logistic regression on a ball of bounded radius reaches tilde{O}(OPT) misclassification risk. Our techniques also show for any well-behaved distribution, regardless of radial Lipschitzness, we can overcome the Omega(sqrt{OPT}) lower bound for logistic loss simply at the cost of one additional convex optimization step involving the hinge loss and attain tilde{O}(OPT) misclassification risk. This two-step convex optimization algorithm is simpler than previous methods obtaining this guarantee, all of which require solving O(log(1/OPT)) minimization problems.
[ Room 301 - 303 ]
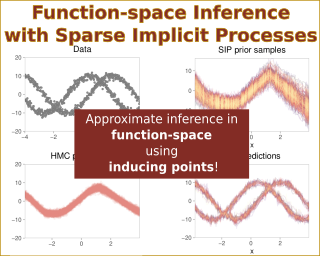
Implicit Processes (IPs) represent a flexible framework that can be used to describe a wide variety of models, from Bayesian neural networks, neural samplers and data generators to many others. IPs also allow for approximate inference in function-space. This change of formulation solves intrinsic degenerate problems of parameter-space approximate inference concerning the high number of parameters and their strong dependencies in large models. For this, previous works in the literature have attempted to employ IPs both to set up the prior and to approximate the resulting posterior. However, this has proven to be a challenging task. Existing methods that can tune the prior IP result in a Gaussian predictive distribution, which fails to capture important data patterns. By contrast, methods producing flexible predictive distributions by using another IP to approximate the posterior process cannot tune the prior IP to the observed data. We propose here the first method that can accomplish both goals. For this, we rely on an inducing-point representation of the prior IP, as often done in the context of sparse Gaussian processes. The result is a scalable method for approximate inference with IPs that can tune the prior IP parameters to the data, and that provides accurate …
[ Room 327 - 329 ]
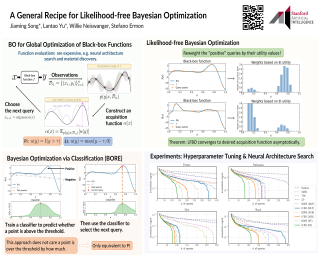
The acquisition function, a critical component in Bayesian optimization (BO), can often be written as the expectation of a utility function under a surrogate model. However, to ensure that acquisition functions are tractable to optimize, restrictions must be placed on the surrogate model and utility function. To extend BO to a broader class of models and utilities, we propose likelihood-free BO (LFBO), an approach based on likelihood-free inference. LFBO directly models the acquisition function without having to separately perform inference with a probabilistic surrogate model. We show that computing the acquisition function in LFBO can be reduced to optimizing a weighted classification problem, which extends an existing likelihood-free density ratio estimation method related to probability of improvement (PI). By choosing the utility function for expected improvement (EI), LFBO outperforms the aforementioned method, as well as various state-of-the-art black-box optimization methods on several real-world optimization problems. LFBO can also leverage composite structures of the objective function, which further improves its regret by several orders of magnitude.
[ Room 310 ]

Decision trees and random forests (RF) are a cornerstone of modern machine learning practice. Due to their tendency to overfit, trees are typically regularized by a variety of techniques that modify their structure (e.g. pruning). We introduce Hierarchical Shrinkage (HS), a post-hoc algorithm which regularizes the tree not by altering its structure, but by shrinking the prediction over each leaf toward the sample means over each of its ancestors, with weights depending on a single regularization parameter and the number of samples in each ancestor. Since HS is a post-hoc method, it is extremely fast, compatible with any tree-growing algorithm and can be used synergistically with other regularization techniques. Extensive experiments over a wide variety of real-world datasets show that HS substantially increases the predictive performance of decision trees even when used in conjunction with other regularization techniques. Moreover, we find that applying HS to individual trees in a RF often improves its accuracy and interpretability by simplifying and stabilizing decision boundaries and SHAP values. We further explain HS by showing that it to be equivalent to ridge regression on a basis that is constructed of decision stumps associated to the internal nodes of a tree. All code and models …
[ Ballroom 3 & 4 ]
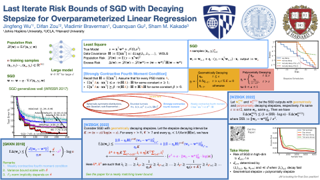
Stochastic gradient descent (SGD) has been shown to generalize well in many deep learning applications. In practice, one often runs SGD with a geometrically decaying stepsize, i.e., a constant initial stepsize followed by multiple geometric stepsize decay, and uses the last iterate as the output. This kind of SGD is known to be nearly minimax optimal for classical finite-dimensional linear regression problems (Ge et al., 2019). However, a sharp analysis for the last iterate of SGD in the overparameterized setting is still open. In this paper, we provide a problem-dependent analysis on the last iterate risk bounds of SGD with decaying stepsize, for (overparameterized) linear regression problems. In particular, for last iterate SGD with (tail) geometrically decaying stepsize, we prove nearly matching upper and lower bounds on the excess risk. Moreover, we provide an excess risk lower bound for last iterate SGD with polynomially decaying stepsize and demonstrate the advantage of geometrically decaying stepsize in an instance-wise manner, which complements the minimax rate comparison made in prior work.
[ Room 301 - 303 ]

Visual systems of primates are the gold standard of robust perception. There is thus a general belief that mimicking the neural representations that underlie those systems will yield artificial visual systems that are adversarially robust. In this work,we develop a method for performing adversarial visual attacks directly on primate brain activity. We then leverage this method to demonstrate that the above-mentioned belief might not be well-founded. Specifically, we report that the biological neurons that make up visual systems of primates exhibit susceptibility to adversarial perturbations that is comparable in magnitude to existing (robustly trained) artificial neural networks.
[ Room 309 ]
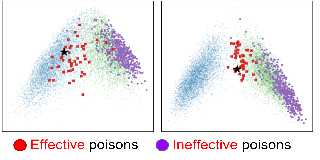
Data poisoning causes misclassification of test time target examples, by injecting maliciously crafted samples in the training data. Existing defenses are often effective only against a specific type of targeted attack, significantly degrade the generalization performance, or are prohibitive for standard deep learning pipelines. In this work, we propose an efficient defense mechanism that significantly reduces the success rate of various data poisoning attacks, and provides theoretical guarantees for the performance of the model. Targeted attacks work by adding bounded perturbations to a randomly selected subset of training data to match the targets’ gradient or representation. We show that: (i) under bounded perturbations, only a number of poisons can be optimized to have a gradient that is close enough to that of the target and make the attack successful; (ii) such effective poisons move away from their original class and get isolated in the gradient space; (iii) dropping examples in low-density gradient regions during training can successfully eliminate the effective poisons, and guarantees similar training dynamics to that of training on full data. Our extensive experiments show that our method significantly decreases the success rate of state-of-the-art targeted attacks, including Gradient Matching and Bullseye Polytope, and easily scales to large …
[ Ballroom 1 & 2 ]

[ Hall G ]
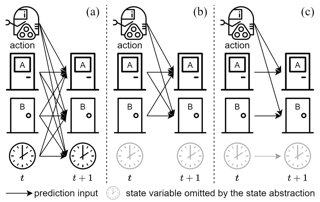
Learning dynamics models accurately is an important goal for Model-Based Reinforcement Learning (MBRL), but most MBRL methods learn a dense dynamics model which is vulnerable to spurious correlations and therefore generalizes poorly to unseen states. In this paper, we introduce Causal Dynamics Learning for Task-Independent State Abstraction (CDL), which first learns a theoretically proved causal dynamics model that removes unnecessary dependencies between state variables and the action, thus generalizing well to unseen states. A state abstraction can then be derived from the learned dynamics, which not only improves sample efficiency but also applies to a wider range of tasks than existing state abstraction methods. Evaluated on two simulated environments and downstream tasks, both the dynamics model and policies learned by the proposed method generalize well to unseen states and the derived state abstraction improves sample efficiency compared to learning without it.
[ Room 307 ]

[ Hall F ]
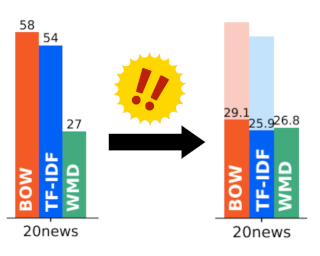
The word mover's distance (WMD) is a fundamental technique for measuring the similarity of two documents. As the crux of WMD, it can take advantage of the underlying geometry of the word space by employing an optimal transport formulation. The original study on WMD reported that WMD outperforms classical baselines such as bag-of-words (BOW) and TF-IDF by significant margins in various datasets. In this paper, we point out that the evaluation in the original study could be misleading. We re-evaluate the performances of WMD and the classical baselines and find that the classical baselines are competitive with WMD if we employ an appropriate preprocessing, i.e., L1 normalization. In addition, we introduce an analogy between WMD and L1-normalized BOW and find that not only the performance of WMD but also the distance values resemble those of BOW in high dimensional spaces.
[ Room 318 - 320 ]

Most of the existing methods for estimating the local intrinsic dimension of a data distribution do not scale well to high dimensional data. Many of them rely on a non-parametric nearest neighbours approach which suffers from the curse of dimensionality. We attempt to address that challenge by proposing a novel approach to the problem: Local Intrinsic Dimension estimation using approximate Likelihood (LIDL).Our method relies on an arbitrary density estimation method as its subroutine, and hence tries to sidestep the dimensionality challenge by making use of the recent progress in parametric neural methods for likelihood estimation.We carefully investigate the empirical properties of the proposed method, compare them with our theoretical predictions, show that LIDL yields competitive results on the standard benchmarks for this problem, and that it scales to thousands of dimensions. What is more, we anticipate this approach to improve further with the continuing advances in the density estimation literature.
[ Hall G ]
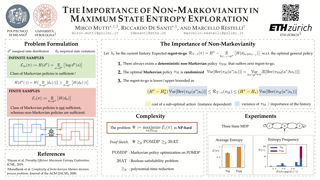
In the maximum state entropy exploration framework, an agent interacts with a reward-free environment to learn a policy that maximizes the entropy of the expected state visitations it is inducing. Hazan et al. (2019) noted that the class of Markovian stochastic policies is sufficient for the maximum state entropy objective, and exploiting non-Markovianity is generally considered pointless in this setting. In this paper, we argue that non-Markovianity is instead paramount for maximum state entropy exploration in a finite-sample regime. Especially, we recast the objective to target the expected entropy of the induced state visitations in a single trial. Then, we show that the class of non-Markovian deterministic policies is sufficient for the introduced objective, while Markovian policies suffer non-zero regret in general. However, we prove that the problem of finding an optimal non-Markovian policy is NP-hard. Despite this negative result, we discuss avenues to address the problem in a tractable way and how non-Markovian exploration could benefit the sample efficiency of online reinforcement learning in future works.
[ Room 307 ]

Since reinforcement learning algorithms are notoriously data-intensive, the task of sampling observations from the environment is usually split across multiple agents. However, transferring these observations from the agents to a central location can be prohibitively expensive in terms of the communication cost, and it can also compromise the privacy of each agent's local behavior policy. In this paper, we consider a federated reinforcement learning framework where multiple agents collaboratively learn a global model, without sharing their individual data and policies. Each agent maintains a local copy of the model and updates it using locally sampled data. Although having N agents enables the sampling of N times more data, it is not clear if it leads to proportional convergence speedup. We propose federated versions of on-policy TD, off-policy TD and Q-learning, and analyze their convergence. For all these algorithms, to the best of our knowledge, we are the first to consider Markovian noise and multiple local updates, and prove a linear convergence speedup with respect to the number of agents. To obtain these results, we show that federated TD and Q-learning are special cases of a general framework for federated stochastic approximation with Markovian noise, and we leverage this framework to …
[ Ballroom 3 & 4 ]

[ Room 309 ]

This paper demonstrates how to recover causal graphs from the score of the data distribution in non-linear additive (Gaussian) noise models. Using score matching algorithms as a building block, we show how to design a new generation of scalable causal discovery methods. To showcase our approach, we also propose a new efficient method for approximating the score's Jacobian, enabling to recover the causal graph. Empirically, we find that the new algorithm, called SCORE, is competitive with state-of-the-art causal discovery methods while being significantly faster.
[ Ballroom 1 & 2 ]
[ Room 327 - 329 ]

We propose efficient random features for approximating a new and rich class of kernel functions that we refer to as Generalized Zonal Kernels (GZK). Our proposed GZK family, generalizes the zonal kernels (i.e., dot-product kernels on the unit sphere) by introducing radial factors in the Gegenbauer series expansion of these kernel functions. The GZK class of kernels includes a wide range of ubiquitous kernel functions such as the entirety of dot-product kernels as well as the Gaussian and the recently introduced Neural Tangent kernels. Interestingly, by exploiting the reproducing property of the Gegenbauer (Zonal) Harmonics, we can construct efficient random features for the GZK family based on randomly oriented Gegenbauer harmonics. We prove subspace embedding guarantees for our Gegenbauer features which ensures that our features can be used for approximately solving learning problems such as kernel k-means clustering, kernel ridge regression, etc. Empirical results show that our proposed features outperform recent kernel approximation methods.
[ Room 318 - 320 ]

This work develops mixup for graph data. Mixup has shown superiority in improving the generalization and robustness of neural networks by interpolating features and labels between two random samples. Traditionally, Mixup can work on regular, grid-like, and Euclidean data such as image or tabular data. However, it is challenging to directly adopt Mixup to augment graph data because different graphs typically: 1) have different numbers of nodes; 2) are not readily aligned; and 3) have unique typologies in non-Euclidean space. To this end, we propose G-Mixup to augment graphs for graph classification by interpolating the generator (i.e., graphon) of different classes of graphs. Specifically, we first use graphs within the same class to estimate a graphon. Then, instead of directly manipulating graphs, we interpolate graphons of different classes in the Euclidean space to get mixed graphons, where the synthetic graphs are generated through sampling based on the mixed graphons. Extensive experiments show that G-Mixup substantially improves the generalization and robustness of GNNs.
[ Room 310 ]
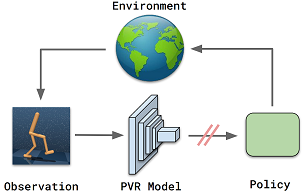
Recent years have seen the emergence of pre-trained representations as a powerful abstraction for AI applications in computer vision, natural language, and speech. However, policy learning for control is still dominated by a tabula-rasa learning paradigm, with visuo-motor policies often trained from scratch using data from deployment environments. In this context, we revisit and study the role of pre-trained visual representations for control, and in particular representations trained on large-scale computer vision datasets. Through extensive empirical evaluation in diverse control domains (Habitat, DeepMind Control, Adroit, Franka Kitchen), we isolate and study the importance of different representation training methods, data augmentations, and feature hierarchies. Overall, we find that pre-trained visual representations can be competitive or even better than ground-truth state representations to train control policies. This is in spite of using only out-of-domain data from standard vision datasets, without any in-domain data from the deployment environments.
[ Room 301 - 303 ]

Developing architectures suitable for modeling raw audio is a challenging problem due to the high sampling rates of audio waveforms. Standard sequence modeling approaches like RNNs and CNNs have previously been tailored to fit the demands of audio, but the resultant architectures make undesirable computational tradeoffs and struggle to model waveforms effectively. We propose SaShiMi, a new multi-scale architecture for waveform modeling built around the recently introduced S4 model for long sequence modeling. We identify that S4 can be unstable during autoregressive generation, and provide a simple improvement to its parameterization by drawing connections to Hurwitz matrices. SaShiMi yields state-of-the-art performance for unconditional waveform generation in the autoregressive setting. Additionally, SaShiMi improves non-autoregressive generation performance when used as the backbone architecture for a diffusion model. Compared to prior architectures in the autoregressive generation setting, SaShiMi generates piano and speech waveforms which humans find more musical and coherent respectively, e.g. 2× better mean opinion scores than WaveNet on an unconditional speech generation task. On a music generation task, SaShiMi outperforms WaveNet on density estimation and speed at both training and inference even when using 3× fewer parameters
[ Hall F ]

Deep learning models are able to approximate one specific dynamical system but struggle at learning generalisable dynamics, where dynamical systems obey the same laws of physics but contain different numbers of elements (e.g., double- and triple-pendulum systems). To relieve this issue, we proposed the Modular Lagrangian Network (ModLaNet), a structural neural network framework with modularity and physical inductive bias. This framework models the energy of each element using modularity and then construct the target dynamical system via Lagrangian mechanics. Modularity is beneficial for reusing trained networks and reducing the scale of networks and datasets. As a result, our framework can learn from the dynamics of simpler systems and extend to more complex ones, which is not feasible using other relevant physics-informed neural networks. We examine our framework for modelling double-pendulum or three-body systems with small training datasets, where our models achieve the best data efficiency and accuracy performance compared with counterparts. We also reorganise our models as extensions to model multi-pendulum and multi-body systems, demonstrating the intriguing reusable feature of our framework.
[ Ballroom 3 & 4 ]
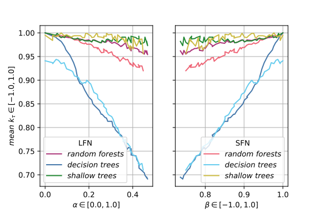
Label Ranking (LR) corresponds to the problem of learning a hypothesis that maps features to rankings over a finite set of labels. We adopt a nonparametric regression approach to LR and obtain theoretical performance guarantees for this fundamental practical problem. We introduce a generative model for Label Ranking, in noiseless and noisy nonparametric regression settings, and provide sample complexity bounds for learning algorithms in both cases. In the noiseless setting, we study the LR problem with full rankings and provide computationally efficient algorithms using decision trees and random forests in the high-dimensional regime. In the noisy setting, we consider the more general cases of LR with incomplete and partial rankings from a statistical viewpoint and obtain sample complexity bounds using the One-Versus-One approach of multiclass classification. Finally, we complement our theoretical contributions with experiments, aiming to understand how the input regression noise affects the observed output.
[ Room 307 ]

Interior point methods (IPMs) are a common approach for solving linear programs (LPs) with strong theoretical guarantees and solid empirical performance. The time complexity of these methods is dominated by the cost of solving a linear system of equations at each iteration. In common applications of linear programming, particularly in machine learning and scientific computing, the size of this linear system can become prohibitively large, requiring the use of iterative solvers, which provide an approximate solution to the linear system. However, approximately solving the linear system at each iteration of an IPM invalidates the theoretical guarantees of common IPM analyses. To remedy this, we theoretically and empirically analyze (slightly modified) predictor-corrector IPMs when using approximate linear solvers: our approach guarantees that, when certain conditions are satisfied, the number of IPM iterations does not increase and that the final solution remains feasible. We also provide practical instantiations of approximate linear solvers that satisfy these conditions for special classes of constraint matrices using randomized linear algebra.
[ Hall G ]
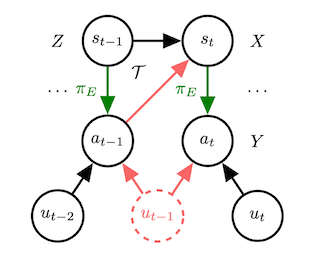
We develop algorithms for imitation learning from policy data that was corrupted by temporally correlated noise in expert actions. When noise affects multiple timesteps of recorded data, it can manifest as spurious correlations between states and actions that a learner might latch on to, leading to poor policy performance. To break up these spurious correlations, we apply modern variants of the instrumental variable regression (IVR) technique of econometrics, enabling us to recover the underlying policy without requiring access to an interactive expert. In particular, we present two techniques, one of a generative-modeling flavor (DoubIL) that can utilize access to a simulator, and one of a game-theoretic flavor (ResiduIL) that can be run entirely offline. We find both of our algorithms compare favorably to behavioral cloning on simulated control tasks.
[ Room 310 ]
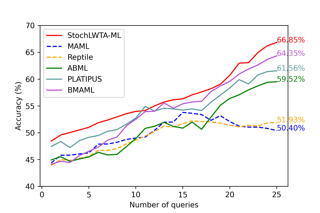
This work addresses meta-learning (ML) by considering deep networks with stochastic local winner-takes-all (LWTA) activations. This type of network units results in sparse representations from each model layer, as the units are organized into blocks where only one unit generates a non-zero output. The main operating principle of the introduced units rely on stochastic principles, as the network performs posterior sampling over competing units to select the winner. Therefore, the proposed networks are explicitly designed to extract input data representations of sparse stochastic nature, as opposed to the currently standard deterministic representation paradigm. Our approach produces state-of-the-art predictive accuracy on few-shot image classification and regression experiments, as well as reduced predictive error on an active learning setting; these improvements come with an immensely reduced computational cost. Code is available at: https://github.com/Kkalais/StochLWTA-ML
[ Room 318 - 320 ]

Maximizing a monotone k-submodular function subject to cardinality constraints is a general model for several applications ranging from influence maximization with multiple products to sensor placement with multiple sensor types and online ad allocation. Due to the large problem scale in many applications and the online nature of ad allocation, a need arises for algorithms that process elements in a streaming fashion and possibly make online decisions. In this work, we develop a new streaming algorithm for maximizing a monotone k-submodular function subject to a per-coordinate cardinality constraint attaining an approximation guarantee close to the state of the art guarantee in the offline setting. Though not typical for streaming algorithms, our streaming algorithm also readily applies to the online setting with free disposal. Our algorithm is combinatorial and enjoys fast running time and small number of function evaluations. Furthermore, its guarantee improves as the cardinality constraints get larger, which is especially suited for the large scale applications. For the special case of maximizing a submodular function with large budgets, our combinatorial algorithm matches the guarantee of the state-of-the-art continuous algorithm, which requires significantly more time and function evaluations.
[ Room 327 - 329 ]
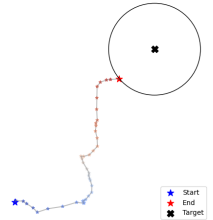
We introduce a method for policy improvement that interpolates between the greedy approach of value-based reinforcement learning (RL) and the full planning approach typical of model-based RL. The new method builds on the concept of a geometric horizon model (GHM, also known as a \gamma-model), which models the discounted state-visitation distribution of a given policy. We show that we can evaluate any non-Markov policy that switches between a set of base Markov policies with fixed probability by a careful composition of the base policy GHMs, without any additional learning. We can then apply generalised policy improvement (GPI) to collections of such non-Markov policies to obtain a new Markov policy that will in general outperform its precursors. We provide a thorough theoretical analysis of this approach, develop applications to transfer and standard RL, and empirically demonstrate its effectiveness over standard GPI on a challenging deep RL continuous control task. We also provide an analysis of GHM training methods, proving a novel convergence result regarding previously proposed methods and showing how to train these models stably in deep RL settings.
[ Ballroom 3 & 4 ]

[ Room 301 - 303 ]

We study representation learning for Offline Reinforcement Learning (RL), focusing on the important task of Offline Policy Evaluation (OPE). Recent work shows that, in contrast to supervised learning, realizability of the Q-function is not enough for learning it. Two sufficient conditions for sample-efficient OPE are Bellman completeness and coverage. Prior work often assumes that representations satisfying these conditions are given, with results being mostly theoretical in nature. In this work, we propose BCRL, which directly learns from data an approximately linear Bellman complete representation with good coverage. With this learned representation, we perform OPE using Least Square Policy Evaluation (LSPE) with linear functions in our learned representation. We present an end-to-end theoretical analysis, showing that our two-stage algorithm enjoys polynomial sample complexity provided some representation in the rich class considered is linear Bellman complete. Empirically, we extensively evaluate our algorithm on challenging, image-based continuous control tasks from the Deepmind Control Suite. We show our representation enables better OPE compared to previous representation learning methods developed for off-policy RL (e.g., CURL, SPR). BCRL achieve competitive OPE error with the state-of-the-art method Fitted Q-Evaluation (FQE), and beats FQE when evaluating beyond the initial state distribution. Our ablations show that both linear Bellman …
[ Room 309 ]

[ Room 307 ]

Recent work highlights the role of causality in designing equitable decision-making algorithms. It is not immediately clear, however, how existing causal conceptions of fairness relate to one another, or what the consequences are of using these definitions as design principles. Here, we first assemble and categorize popular causal definitions of algorithmic fairness into two broad families: (1) those that constrain the effects of decisions on counterfactual disparities; and (2) those that constrain the effects of legally protected characteristics, like race and gender, on decisions. We then show, analytically and empirically, that both families of definitions \emph{almost always}---in a measure theoretic sense---result in strongly Pareto dominated decision policies, meaning there is an alternative, unconstrained policy favored by every stakeholder with preferences drawn from a large, natural class. For example, in the case of college admissions decisions, policies constrained to satisfy causal fairness definitions would be disfavored by every stakeholder with neutral or positive preferences for both academic preparedness and diversity.Indeed, under a prominent definition of causal fairness, we prove the resulting policies require admitting all students with the same probability, regardless of academic qualifications or group membership. Our results highlight formal limitations and potential adverse consequences of common mathematical notions of …
[ Ballroom 1 & 2 ]

We consider unsupervised domain adaptation (UDA), where labeled data from a source domain (e.g., photos) and unlabeled data from a target domain (e.g., sketches) are used to learn a classifier for the target domain. Conventional UDA methods (e.g., domain adversarial training) learn domain-invariant features to generalize from the source domain to the target domain. In this paper, we show that contrastive pre-training, which learns features on unlabeled source and target data and then fine-tunes on labeled source data, is competitive with strong UDA methods. However, we find that contrastive pre-training does not learn domain-invariant features, diverging from conventional UDA intuitions. We show theoretically that contrastive pre-training can learn features that vary subtantially across domains but still generalize to the target domain, by disentangling domain and class information. We empirically validate our theory on benchmark vision datasets.
[ Hall G ]
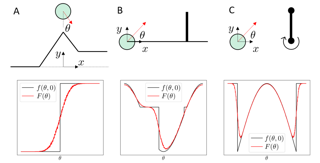
[ Hall F ]

Compositional generalization is a critical ability in learning and decision-making. We focus on the setting of reinforcement learning in object-oriented environments to study compositional generalization in world modeling. We (1) formalize the compositional generalization problem with an algebraic approach and (2) study how a world model can achieve that. We introduce a conceptual environment, Object Library, and two instances, and deploy a principled pipeline to measure the generalization ability. Motivated by the formulation, we analyze several methods with exact or no compositional generalization ability using our framework, and design a differentiable approach, Homomorphic Object-oriented World Model (HOWM), that achieves soft but more efficient compositional generalization.
[ Room 310 ]

How do we compare between hypotheses that are entirely consistent with observations? The marginal likelihood (aka Bayesian evidence), which represents the probability of generating our observations from a prior, provides a distinctive approach to this foundational question, automatically encoding Occam's razor. Although it has been observed that the marginal likelihood can overfit and is sensitive to prior assumptions, its limitations for hyperparameter learning and discrete model comparison have not been thoroughly investigated. We first revisit the appealing properties of the marginal likelihood for learning constraints and hypothesis testing. We then highlight the conceptual and practical issues in using the marginal likelihood as a proxy for generalization. Namely, we show how marginal likelihood can be negatively correlated with generalization, with implications for neural architecture search, and can lead to both underfitting and overfitting in hyperparameter learning. We provide a partial remedy through a conditional marginal likelihood, which we show is more aligned with generalization, and practically valuable for large-scale hyperparameter learning, such as in deep kernel learning.
[ Room 318 - 320 ]
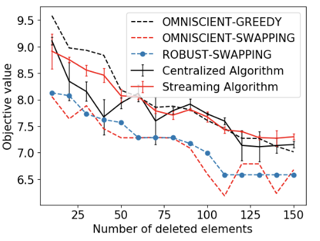
[ Room 301 - 303 ]
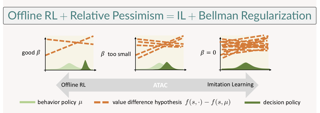
We propose Adversarially Trained Actor Critic (ATAC), a new model-free algorithm for offline reinforcement learning (RL) under insufficient data coverage, based on the concept of relative pessimism. ATAC is designed as a two-player Stackelberg game framing of offline RL: A policy actor competes against an adversarially trained value critic, who finds data-consistent scenarios where the actor is inferior to the data-collection behavior policy. We prove that, when the actor attains no regret in the two-player game, running ATAC produces a policy that provably 1) outperforms the behavior policy over a wide range of hyperparameters that control the degree of pessimism, and 2) competes with the best policy covered by data with appropriately chosen hyperparameters. Compared with existing works, notably our framework offers both theoretical guarantees for general function approximation and a deep RL implementation scalable to complex environments and large datasets. In the D4RL benchmark, ATAC consistently outperforms state-of-the-art offline RL algorithms on a range of continuous control tasks.
[ Ballroom 3 & 4 ]

We study a variant of a recently introduced min-max optimization framework where the max-player is constrained to update its parameters in a greedy manner until it reaches a first-order stationary point. Our equilibrium definition for this framework depends on a proposal distribution which the min-player uses to choose directions in which to update its parameters. We show that, given a smooth and bounded nonconvex-nonconcave objective function, access to any proposal distribution for the min-player’s updates, and stochastic gradient oracle for the max-player, our algorithm converges to the aforementioned approximate local equilibrium in a number of iterations that does not depend on the dimension. The equilibrium point found by our algorithm depends on the proposal distribution, and when applying our algorithm to train GANs we choose the proposal distribution to be a distribution of stochastic gradients. We empirically evaluate our algorithm on challenging nonconvex-nonconcave test-functions and loss functions arising in GAN training. Our algorithm converges on these test functions and, when used to train GANs, trains stably on synthetic and real-world datasets and avoids mode collapse.
[ Room 327 - 329 ]
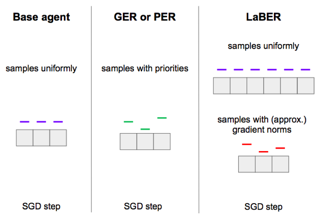
Several algorithms have been proposed to sample non-uniformly the replay buffer of deep Reinforcement Learning (RL) agents to speed-up learning, but very few theoretical foundations of these sampling schemes have been provided. Among others, Prioritized Experience Replay appears as a hyperparameter sensitive heuristic, even though it can provide good performance. In this work, we cast the replay buffer sampling problem as an importance sampling one for estimating the gradient. This allows deriving the theoretically optimal sampling distribution, yielding the best theoretical convergence speed.Elaborating on the knowledge of the ideal sampling scheme, we exhibit new theoretical foundations of Prioritized Experience Replay. The optimal sampling distribution being intractable, we make several approximations providing good results in practice and introduce, among others, LaBER (Large Batch Experience Replay), an easy-to-code and efficient method for sampling the replay buffer. LaBER, which can be combined with Deep Q-Networks, distributional RL agents or actor-critic methods, yields improved performance over a diverse range of Atari games and PyBullet environments, compared to the base agent it is implemented on and to other prioritization schemes.
[ Room 309 ]
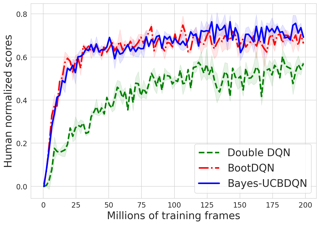
[ Room 318 - 320 ]

Strategic classification studies learning in settings where self-interested users can strategically modify their features to obtain favorable predictive outcomes. A key working assumption, however, is that “favorable” always means “positive”; this may be appropriate in some applications (e.g., loan approval), but reduces to a fairly narrow view of what user interests can be. In this work we argue for a broader perspective on what accounts for strategic user behavior, and propose and study a flexible model of generalized strategic classification. Our generalized model subsumes most current models but includes other novel settings; among these, we identify and target one intriguing sub-class of problems in which the interests of users and the system are aligned. This setting reveals a surprising fact: that standard max-margin losses are ill-suited for strategic inputs. Returning to our fully generalized model, we propose a novel max-margin framework for strategic learning that is practical and effective, and which we analyze theoretically. We conclude with a set of experiments that empirically demonstrate the utility of our approach.
[ Room 310 ]

We introduce a novel framework for optimization based on energy-conserving Hamiltonian dynamics in a strongly mixing (chaotic) regime and establish its key properties analytically and numerically. The prototype is a discretization of Born-Infeld dynamics, with a squared relativistic speed limit depending on the objective function. This class of frictionless, energy-conserving optimizers proceeds unobstructed until slowing naturally near the minimal loss, which dominates the phase space volume of the system. Building from studies of chaotic systems such as dynamical billiards, we formulate a specific algorithm with good performance on machine learning and PDE-solving tasks, including generalization. It cannot stop at a high local minimum, an advantage in non-convex loss functions, and proceeds faster than GD+momentum in shallow valleys.
[ Hall G ]
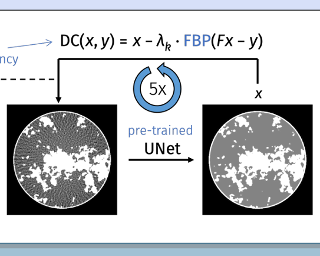
This work is concerned with the following fundamental question in scientific machine learning: Can deep-learning-based methods solve noise-free inverse problems to near-perfect accuracy? Positive evidence is provided for the first time, focusing on a prototypical computed tomography (CT) setup. We demonstrate that an iterative end-to-end network scheme enables reconstructions close to numerical precision, comparable to classical compressed sensing strategies. Our results build on our winning submission to the recent AAPM DL-Sparse-View CT Challenge. Its goal was to identify the state-of-the-art in solving the sparse-view CT inverse problem with data-driven techniques. A specific difficulty of the challenge setup was that the precise forward model remained unknown to the participants. Therefore, a key feature of our approach was to initially estimate the unknown fanbeam geometry in a data-driven calibration step. Apart from an in-depth analysis of our methodology, we also demonstrate its state-of-the-art performance on the open-access real-world dataset LoDoPaB CT.
[ Room 327 - 329 ]

Present-day federated learning (FL) systems deployed over edge networks consists of a large number of workers with high degrees of heterogeneity in data and/or computing capabilities, which call for flexible worker participation in terms of timing, effort, data heterogeneity, etc. To satisfy the need for flexible worker participation, we consider a new FL paradigm called ``Anarchic Federated Learning'' (AFL) in this paper. In stark contrast to conventional FL models, each worker in AFL has the freedom to choose i) when to participate in FL, and ii) the number of local steps to perform in each round based on its current situation (e.g., battery level, communication channels, privacy concerns). However, such chaotic worker behaviors in AFL impose many new open questions in algorithm design. In particular, it remains unclear whether one could develop convergent AFL training algorithms, and if yes, under what conditions and how fast the achievable convergence speed is. Toward this end, we propose two Anarchic Federated Averaging (AFA) algorithms with two-sided learning rates for both cross-device and cross-silo settings, which are named AFA-CD and AFA-CS, respectively. Somewhat surprisingly, we show that, under mild anarchic assumptions, both AFL algorithms achieve the best known convergence rate as the state-of-the-art algorithms …
[ Ballroom 3 & 4 ]

Recently, several universal methods have been proposed for online convex optimization, and attain minimax rates for multiple types of convex functions simultaneously. However, they need to design and optimize one surrogate loss for each type of functions, making it difficult to exploit the structure of the problem and utilize existing algorithms. In this paper, we propose a simple strategy for universal online convex optimization, which avoids these limitations. The key idea is to construct a set of experts to process the original online functions, and deploy a meta-algorithm over the linearized losses to aggregate predictions from experts. Specifically, the meta-algorithm is required to yield a second-order bound with excess losses, so that it can leverage strong convexity and exponential concavity to control the meta-regret. In this way, our strategy inherits the theoretical guarantee of any expert designed for strongly convex functions and exponentially concave functions, up to a double logarithmic factor. As a result, we can plug in off-the-shelf online solvers as black-box experts to deliver problem-dependent regret bounds. For general convex functions, it maintains the minimax optimality and also achieves a small-loss bound.
[ Room 307 ]

We present an analytical policy update rule that is independent of parametric function approximators. The policy update rule is suitable for optimizing general stochastic policies and has a monotonic improvement guarantee. It is derived from a closed-form solution to trust-region optimization using calculus of variation, following a new theoretical result that tightens existing bounds for policy improvement using trust-region methods. The update rule builds a connection between policy search methods and value function methods. Moreover, off-policy reinforcement learning algorithms can be derived from the update rule since it does not need to compute integration over on-policy states. In addition, the update rule extends immediately to cooperative multi-agent systems when policy updates are performed by one agent at a time.
[ Hall F ]

Recent investigations in noise contrastive estimation suggest, both empirically as well as theoretically, that while having more negative samples'' in the contrastive loss improves downstream classification performance initially, beyond a threshold, it hurts downstream performance due to acollision-coverage'' trade-off. But is such a phenomenon inherent in contrastive learning?We show in a simple theoretical setting, where positive pairs are generated by sampling from the underlying latent class (introduced by Saunshi et al. (ICML 2019)), that the downstream performance of the representation optimizing the (population) contrastive loss in fact does not degrade with the number of negative samples. Along the way, we give a structural characterization of the optimal representation in our framework, for noise contrastive estimation. We also provide empirical support for our theoretical results on CIFAR-10 and CIFAR-100 datasets.
[ Room 301 - 303 ]
We tackle, in the multiple-play bandit setting, the online ranking problem of assigning L items to K predefined positions on a web page in order to maximize the number of user clicks. We propose a generic algorithm, UniRank, that tackles state-of-the-art click models. The regret bound of this algorithm is a direct consequence of the pseudo-unimodality property of the bandit setting with respect to a graph where nodes are ordered sets of indistinguishable items. The main contribution of UniRank is its O(L/∆ logT) regret for T consecutive assignments, where ∆ relates to the reward-gap between two items. This regret bound is based on the usually implicit condition that two items may not have the same attractiveness. Experiments against state-of-the-art learning algorithms specialized or not for different click models, show that our method has better regret performance than other generic algorithms on real life and synthetic datasets.
[ Ballroom 1 & 2 ]

A major challenge in studying robustness in deep learning is defining the set of meaningless perturbations to which a given Neural Network (NN) should be invariant. Most work on robustness implicitly uses a human as the reference model to define such perturbations. Our work offers a new view on robustness by using another reference NN to define the set of perturbations a given NN should be invariant to, thus generalizing the reliance on a reference human NN to any NN. This makes measuring robustness equivalent to measuring the extent to which two NNs share invariances, for which we propose a measure called STIR. STIR re-purposes existing representation similarity measures to make them suitable for measuring shared invariances. Using our measure, we are able to gain insights into how shared invariances vary with changes in weight initialization, architecture, loss functions, and training dataset. Our implementation is available at: https://github.com/nvedant07/STIR
[ Room 318 - 320 ]

[ Room 309 ]

Recent work has shown that offline reinforcement learning (RL) can be formulated as a sequence modeling problem~\cite{chen2021decision, janner2021offline} and solved via approaches similar to large-scale language modeling. However, any practical instantiation of RL also involves an online component, where policies pretrained on passive offline datasets are finetuned via task-specific interactions with the environment. We propose Online Decision Transformers (ODT), an RL algorithm based on sequence modeling that blends offline pretraining with online finetuning in a unified framework. Our framework uses sequence-level entropy regularizers in conjunction with autoregressive modeling objectives for sample-efficient exploration and finetuning. Empirically, we show that ODT is competitive with the state-of-the-art in absolute performance on the D4RL benchmark but shows much more significant gains during the finetuning procedure.